Decrement Factor for grounding design 101 easy explanation

Decrement Factor for grounding design

Decrement factor D_f is defined as the ratio of the the rms value of the asymmetrical fault current and the rms symmetrical grounding fault current. I_G is the maximum asymmetrical alternating current flowing from the grounding grid to the surrounding earth, which includes the rms symmetric alternating current, Ig and the decaying DC component i_dc. The DC offset, which means the difference between the symmetrical wave of current and the actual current wave during a transient condition. Mathematically, the actual wave of current can be divided into two parts, a symmetrical alternating current component and a unidirectional (DC) component.

The unidirectional DC component can be of either polarity, but it will not change polarity and it decreases at some predetermined rate. The DC component refers to the DC offset current, which exponentially decays over time.

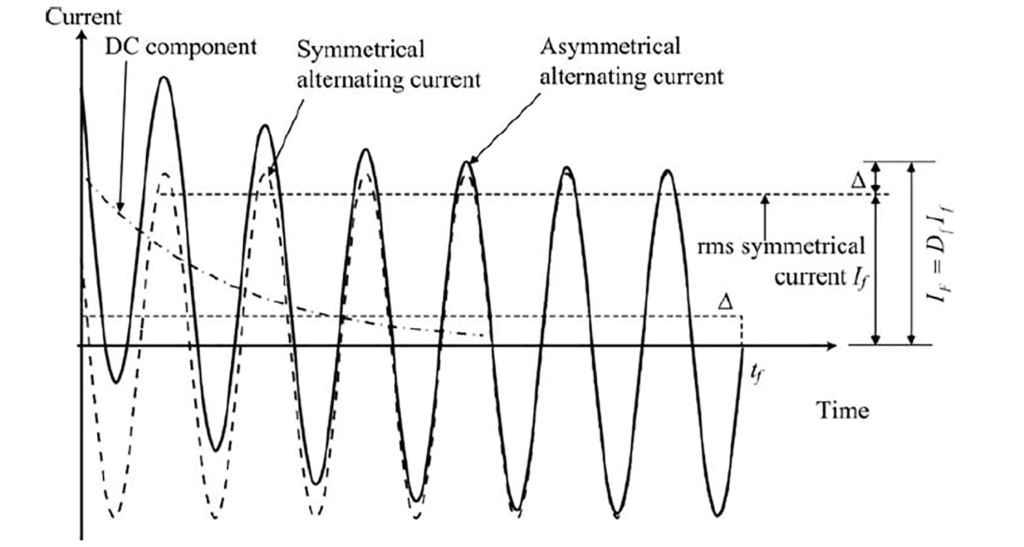
As in the design of a grounding system, the non-symmetrical current component must be taken into account, in order to consider the non-symmetrical fault current wave, which is caused by the DC component in several beginning cycles of the fault current.

The decrement factor D_f should also be considered, which means the rms value of the asymmetrical fault current I_F and the rms symmetrical grounding fault current I_f plus the equivalent DC component ∆, where

$[\Delta = \frac{1}{t_f} \int_0^{t_f} i_{\text{dc}}(t) \, dt]$

Generally, a non-symmetrical fault current is that which includes sub-transient, transient and steady-state AC components and the DC offset current component. The sub-transient and transient AC components and the DC offset current component, which decays exponentially, their rates of attenuation are different.

However, for the sake of simplicity, in IEEE Standard 80-2000 it is assumed that AC component does not decay with time but remains at its initial value. Therefore, a non-symmetrical fault current, that is a periodic function of time, which can be expressed as

$i_f(t) = \sqrt{2} \, U_Y \left[ \sin \left( \omega_t + \alpha - \theta \right) - \exp \left( -\frac{t}{T_a} \right) \sin \left( \alpha - \theta \right) \right]$

where U is the pre fault rms line to neutral voltage (in V), ω is the frequency (in radians in s), α is the voltage angle at current initiation (in radians), θ is the circuit phase angle (in radians), Y is the equivalent AC system admittance (in mhos) and T_a is the time constant of the DC offset component in s; T_a = X/ ωR , where the X and R of the system sub-transient fault impedance in the location of the fault for a given type of fault are used to determine the ratio X/R.

In reality, faults can occur at random times with respect to the voltage wave. The shock contact may occur at any moment after the fault is initiated. Therefore, to determine the most severe condition, it is necessary to assume that the maximum possible DC offset will be present at the moment of an accidental shock contact. When α – θ = – π/2 , putting it in the above Equation, the DC offset component is at the maximum, then Equation changes to

$i_f(t) = \sqrt{2} \, U_Y \left[ -\cos \left( \omega_t \right) - \exp \left( -\frac{t}{T_a} \right) \right]$

The experimental data for heart fibrillation is based on the energy value of a symmetrical sine wave with constant amplitude. But, for a current wave which is non-symmetrical, its equivalent rms value should therefore be determined based on the possible largest time of the accidental shock contact. The effective asymmetrical fault current I_F can be determined by the integration of the above equation squared over the entire duration of fault t_f

$I_F = \sqrt{\frac{1}{t_f} \int_0^{t_f} i_f^2(t) \, dt}$

where I_F is the effective rms value of the non-symmetry current in the whole fault duration and t_f is the duration of fault (in s).

Therefore, from the above two equations:

$I_F = I_f \sqrt{\frac{2}{t_f} \int_0^{t_f} \left[ \exp \left( -\frac{t}{T_a} \right) - \cos (\omega t) \right]^2 \, dt}$

MATHEMATICAL EXPRESSION FOR DECREMENT FACTOR

So, the decrement factor D_f is defined as the ratio of I_F and I_f

D_f= I_F / I_f

$D_f = \sqrt{1 + \frac{T_a}{t_f} \left[ 1 - \exp \left( -\frac{2 t_f}{T_a} \right) \right]}$

Based on this equation, we can calculate the decrement factor corresponding to different X/R ratio and fault durations. We know that X/R is proportional to the time constant L/R and can characterize the attenuation velocity of the DC offset. A large X/R ratio corresponds to a large time constant and a small attenuation velocity. Apparently, the integral value mentioned above is determined by the attenuation velocity of the AC and DC components, thus an accurate solution is extremely complex.

Generally, we can use the typical value of the decrement factor which corresponds to different X/R and fault durations shown in Table below, where the respective frequency is 50Hz. If the duration lies in the middle of two data, a linear interpolation must be used to obtain the respective value.

Table for decrement factor selection

Fault Duration t_𝑓 (s)	Decrement factor D_f
Fault Duration t_𝑓 (s)	X/R = 10	X/R = 20	X/R = 30	X/R = 40
0.01	1.576	1.648	1.675	1.688
0.02	1.462	1.576	1.623	1.648
0.05	1.269	1.417	1.497	1.544
0.1	1.148	1.269	1.355	1.417
0.2	1.077	1.148	1.213	1.269
0.3	1.052	1.101	1.148	1.192
0.4	1.039	1.077	1.113	1.148
0.5	1.031	1.062	1.091	1.120
0.75	1.021	1.042	1.062	1.082
1.0	1.016	1.031	1.047	1.062

If the duration is relatively long, it can be considered that the influence of the DC component is larger than the effect of the AC component’s attenuation offset of the symmetrical component’s maximum effective value at the initialization of the fault. So, when the fault’s duration is more than 30 cycles, the decrement factor is equal to 1.

For multiple electric shocks with very short time intervals, such as those caused by automatic reclosing, in the past the decrement factor used to be calculated by the shortest fault duration, even if the time t used in other parts of the calculation was equal to the sum of the duration of all closely spaced successive shocks.

However, the analysis shows that using the shortest duration to determine the decrement factor and using the longest electric shock duration, or the sum of electric shock withstand duration to determine the body permitted voltage, resulted in too conservative a grounding system design.

Especially for a fault with moderate duration (6–30 cycles), the respective fault current division factor is comparatively large.

Thus, when designing the grounding grid, we should conservatively consider whether to use the longest shock fault duration to estimate personal safety, and the shortest fault duration to determine the decrement factor.

Generally, the use of the shortest clearing time of a primary-side circuit breaker will lead to the largest value of D_f.

The DC transient component or the AC transient component, both depends on the ratio of circuit reactance and resistance X/R. However, the above derivation of the decrement factor does not take the fault path resistance into account, thus the result is higher than the actual value, and the error tends to be safe. If a more accurate calculation is needed, then network analysis should be used.

Decrement Factor for grounding design 101 easy explanation